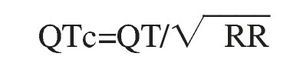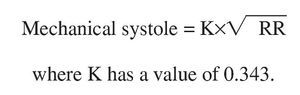The article by Medeiros-Domingo et al1 presents an excellent review of clinical and genetic aspects of long QT syndrome. However, as in almost all recent publications, it attributes the formula for the heart-rate corrected QT interval (QTc) to H.C. Bazett:

As Bazett's actual role in the elaboration of this formula was quite minor, it seems fitting to present a brief historical review of the subject, identifying the contributions of those principally involved.

Mechanical systole duration was a topic of great interest to 19th century pioneers in cardiovascular physiology, and in 1891 A.D. Waller, famous today for his contributions to the development of the electrocardiograph,2 proposed the following expression3 for normal systolic duration:

In 1920, Bazett4 simply adapted this formula to the duration of the electric systole of the heart, the QT interval, and proposed that the normal QT value for a certain heart rate is K´√RR, where K=0.37 for males and 0.4 for females. Thus, to determine if a particular patient has a normal QT interval, his/her QT should be compared with the ideal QT interval derived from Bazett's original formula. This ideal QT interval is that which appears on commonly-used electrocardiogram rulers.

The concept of corrected QT, which is the QT interval that a particular patient would theoretically present with a heart rate of 60 beats/min, and the expression used today to calculate it, erroneously attributed to Bazett, is owed to the forgotten researchers L.M. Taran and N. Szilagyi.5 It is interesting to note that, in classic texts from the 1960s, the role of these authors was clearly recognised and one referred to the "Taran and Szilagyi corrected QT interval6" or the "Bazett formula modified by Taran and Szilagyi.7"

So many years later, is it possible to do them justice and change the name of Bazett's formula to Waller-Bazett-Taran-Szilagyi? Surely not. Force of habit and word economy make it impossible. But if at times, when referring to or hearing about "Bazett's formula," some of us think "and Waller's and Taran's and Szilagyi's," it is probably enough.