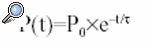Keywords
INTRODUCTION
From a clinical perspective, pulmonary circulation has stimulated much interest among pediatricians due to the frequency and importance of vascular pulmonary changes in congenital cardiopathy, and among cardiologists treating chronic and acute pulmonary artery hypertension (PAH).1 Pulmonary artery hypertension is defined by a mean blood pressure greater than 20 mm Hg at rest or 30 mm Hg during activity at sea-level.2
The pulmonary circuit receives the same blood flow from the heart as the systemic vascular tree and has a similar periodicity. However, there are several structural and physiological differences between the two vascular circuits.3-5 The great arteries fulfill two different but interrelated functions. First, they are low-resistance blood distribution channels that deliver an appropriate blood supply to the peripheral organs. This is called the conduction function and it is related to the static component of the arterial pressure (mean arterial pressure). Second, they buffer pressure oscillations caused by the intermittent nature of the ventricular ejection. This is known as the buffering function (BF) and is related to the pulsatile component (pulse pressure).3,6,7 Owing to the BF, the great arteries store part of the systolic volume during systolic ejection (approximately, 60% under normal conditions) and return it during diastole, losing 15% of the stored energy as heat (dissipated energy).7 This is known as the Windkessel effect, which transforms the pulse flow of the central arteries into a continuous flow required by peripheral tissues. The efficiency of BF is determined by the mechanical properties of the arterial wall (including its geometric characteristics), as these have a protective function on the heart and the arterial system. Thus, it reduces heart work and the development of myocardial tension during systole. It also reduces arterial-parietal pulsatile stress by reducing pressure and flow changes during systole and diastole. Changes in the viscoelastic properties of the arterial walls are due to: a) the mechanical effect of variations in arterial pressure; b) functional changes in vascular tone, and c) structural changes in the arterial wall.3,6-8
When the arterial system becomes more rigid or less distensible in cardiovascular diseases concomitant with PAH, the Windkessel function is reduced. This increases fatigue in the arterial wall and cardiac afterload, causing ventricular hypertrophy and ventriculoarterial uncoupling.9
One of the mechanical properties of the arterial wall used to quantify vascular rigidity10 is elasticity, which has an inverse relationship to compliance in great arteries. Vascular rigidity can also be quantified by pulse wave velocity (PWV).11 The more rigid the vessel, the faster the pressure wave is propagated. Finally, global BF can be characterized by the time constant (τ) extracted from the two-element Windkessel model. In systemic arteries, VSM activation has a protective function, given that it preserves parietal stiffness during the increase in intravascular pressure.12,13
To the best of our knowledge no study has dealt with the in vivo estimation of the pulmonary artery BF. The aim of the present study was to characterize the mechanical properties of the pulmonary artery in vivo with indexes of local parietal stiffness--both dependent on (PWV) and independent from (elasticity) the vascular geometry--as well as with indexes of global parietal stiffness (τ) during the control state and during acute and moderate active and passive PAH.
MATERIAL AND METHODS
Instruments and intervention
Nine merino sheep were anesthetized (26±4.5 kg) by intravenous injection of pentobarbital (35 mg/kg). A polyethylene catheter was placed in the saphenous vein for fluid replacement and delivery of the anesthetic and pharmacological agents. The animals were tracheotomized and ventilated with a positive-pressure ventilator (Drägger SIMV Polyred 201). Oxygen and carbon dioxide partial pressures were monitored. Flow volume and respiratory frequency were set to keep pCO2 between 35 and 45 mm Hg, and pH between 7.35 and 7.4. Arterial pO2 always exceeded 80 mm Hg. The heart was exposed by a thoracotomy through the fourth intercostal space. Once the pericardium was opened, a pressure microtransducer was inserted (Konigsberg Instruments, Inc., Pasadena, CA) through a small incision in the common pulmonary artery wall. A pair of piezoelectric crystals (5 MHz, 3 mm diameter) were sutured to the adventitia of the pulmonary artery wall distal to the microtransducer. The time of the ultrasonic signal (1580 m/s) was converted into distance with a sonomicrometer (Triton Technology Inc. San Diego, CA). The optimal quality of the signal was confirmed by oscilloscope (Tektronix, 465B). This methodology, used in previous studies,10,13,14 allowed us to obtain reliable, reproducible measurements due to the high frequency and linearity of response of the ultrasonic sensors and the microtransducer. A pneumatic occluder was placed around the left pulmonary artery.
Experimental protocol
Following surgical instrumentation, the external diameter and pressure of the pulmonary artery was recorded, under baseline conditions (control steady state), during the occlusion of the left branch of the pulmonary artery and during intravenous infusion of phenylephrine (5 μg/kg/min, Sigma, St. Louis, MO). The return of pressure and diameter values to the control steady state were confirmed between each PAH maneuver.
Because the variations in pressure and diameter were extremely fast, the response of the pulmonary artery wall to the occlusion maneuver only reflects the passive elastic properties intrinsic to the vascular wall (passive PAH). During phenylephrine infusion, instantaneous pressure and diameter variables were monitored until their stabilization 15 to 20 min after beginning infusion. Phenylephrine was chosen to activate VSM because it is a sympathomimetic drug with α1 agonist action that mimics the actions of the sympathetic nervous system15 (active PAH).
All experimental procedures were done according to the ethical criteria and international recommendations on laboratory animals, ratified in Helsinki and revised in 1981 by the American Society of Physiology.16
Data acquisition and determination of elasticity indexes
Pulmonary pressure and diameter was monitored in real time with hardware and software specifically developed in our laboratory (SAMAY MD16).17 Animals were disconnected from the ventilator during data acquisition. The sampling frequency (200 Hz) for data digitalization was at least double the highest frequency component of the pressure and diameter signals spectrum, enabling distortion-free reconstruction. Twenty consecutive beats were analyzed during the three experimental conditions on an IBM PC.
Once the pressure-diameter loop was obtained, the area of hysteresis was reduced to a minimum by increasing the modulus of viscosity (Figure 1). This was done with the Kelvin-Voigt viscoelastic model. Once the purely elastic pressure-diameter relationship was obtained, elasticity was calculated as the first derivative of the mean diastolic pressure (Appendix). Pulse wave velocity was calculated with the Moens-Korteweg equation. The time constant τ was determined with the two-element Windkessel model by fitting an exponential function to the end-diastolic portion of the pulmonary pressure curve (Appendix).
Fig. 1. A: graph of the pressure-diameter loop of the pulmonary artery obtained in the animal model. B: graph of the purely elastic pressure-diameter relation obtained after elimination of the viscous component (solid line), and the elastic pressure-diameter curve based on the logarithmic model (dotted line).
Statistical analysis
All data were expressed as mean±SD (standard deviation). Significance of the differences was tested with ANOVA followed by Student's t test for paired samples. A value of P≤.05 was considered significant.
RESULTS
Hemodynamic data
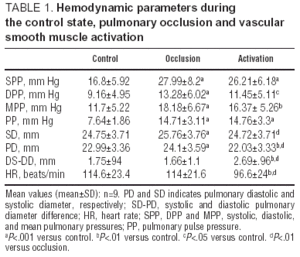
Table 1 shows the hemodynamic parameters (mean±SD) during the three experimental conditions. Systolic, diastolic, mean and pulse pressures were similar during the two PAH states, but significantly higher in comparison to baseline conditions. Systolic diameter increased by 4% (P<.001) during mechanical occlusion of the pulmonary artery, whereas no modification occurred during VSM activation. Diastolic diameter increased significantly during occlusion of the pulmonary artery and decreased when VSM was activated with phenylephrine. Although the increase in pulse pressure was similar during both PAH states, only the pulsatile diameter increased (P<.05) during VSM activation. Finally, heart rate remained unchanged during passive PAH and decreased significantly during phenylephrine infusion.
Elasticity of the arterial wall
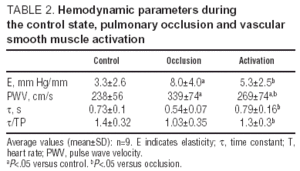
Table 2 summarizes the elasticity indexes during the experimental conditions, showing a significant increase in elasticity during passive PAH. In contrast, elasticity remained unchanged during VSM activation. The increase in PWV during pulmonary artery occlusion and VSM activation was 33±23% and 15±21.6% (P<.05), compared to baseline conditions. τ decreased significantly during passive PAH, whereas it remained unchanged during VSM activation.
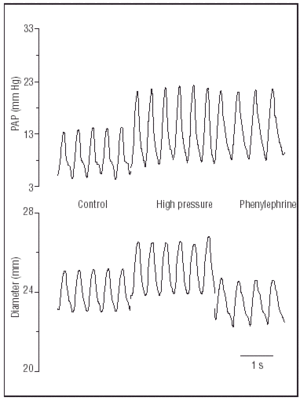
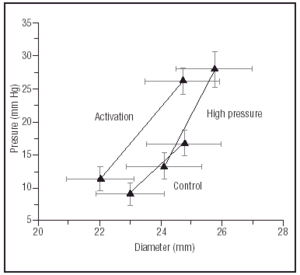
Figure 2 shows representative pressure and diameter tracings during each experimental condition. Vascular smooth muscle activation with phenylephrine prevented dilatation of the arterial wall despite an increase in pressure similar to the pulmonary artery condition. Figure 3 shows mean systolic and diastolic values (mean±SEM) of arterial pressure and diameter during each experimental condition. Vascular smooth muscle activation shifted the pressure-diameter ratio to the left, leading to an isobaric contraction of the arterial wall.
Fig. 2. Pressure and pulmonary external diameter tracing during the control steady state, pulmonary occlusion and vascular smooth muscle activation.
Fig. 3. Diagram showing systolic and diastolic values of pulmonary pressure and diameter in all animals (mean±SEM).
DISCUSSION
The aim of the present study was to estimate the local elastic behavior of the pulmonary artery in vivo by dependent (PWV) and independent (elasticity) indexes of vessel geometry together with a global index of the buffering function (τ), during active and passive acute PAH.
Phenylephrine was used to activate the VSM. This drug can modify the elasticity of the pulmonary artery through a direct effect on the vascular wall or through the distension of the wall secondary to the increase in intravascular pressure generated by pulmonary vasoconstriction. To distinguish between the two effects, arterial pressure was increased by the mechanical occlusion of the left pulmonary artery with a vascular occluder. The elastic behavior of the pulmonary artery wall could thus be compared with and without VSM activation.
After ensuring a similar increase in intravascular arterial pressure during both PAH states (isobaric analysis), elasticity increased significantly due to dilatation of the pulmonary artery only during passive PAH. This was evidenced by an increase in the systolic and diastolic values of the pulmonary diameter, although there were no changes in pulsatile diameter.
The reduction in elasticity observed during VSM activation and the isobaric contraction in diameter may be related to the balance between the increase in peripheral resistance and the reduction in stiffness of the pulmonary artery secondary to the general pulmonary vasoconstriction caused by phenylephrine.18
Consistent with these results, Cox19 demonstrated, in pulmonary artery rings, that VSM activation shifted the stress-strain curve upwards and to the left. When a graph of elasticity as a function of transmural pressure was plotted, the author found that VSM activation caused a significant decrease in elasticity for a constant vascular radius (isometric analysis), both in intralobar and extralobar arteries.
Pulse wave velocity was calculated with the Moens-Korteweg equation to obtain an indirect measure of the parietal stiffness of the pulmonary artery. Our results are in agreement with the values (around 250 cm/s) obtained in dogs and humans for normal pulmonary pressure.3,19-21 During acute arterial occlusion, the increase in PWV may be a purely passive phenomenon due to greater stretching of the vessel, transferring stress from the elastic component of the wall to the collagen. During VSM activation, the smaller increase in PWV (33% vs 15%) delayed the return of the reflex wave, thus preventing an increase in ventricular afterload.2
In light of the characterization of the behavior of the aortic wall by Armentano et al.,10 the isobaric contraction in diameter produced by VSM activation may reduce the participation of collagen, preventing recruitment of collagen fibers that stiffen the vessel wall. Thus, whereas elastin and collagen contribute passively to the elasticity or stiffness of the vessel, the degree of VSM activation seems to actively modulate vascular compliance and PWV, independently of the effect of arterial pressure. This might reduce variations in pulmonary artery impedance, allowing ventriculo-arterial coupling to be preserved in active PAH situations.
In simplified terms, the time constant τ represents the hydraulic arterial load of the cardiac pump. This is the product of total arterial compliance (the pulsatile component of arterial load) and total peripheral resistance (the stationary component of arterial load). τ characterizes the global capacity of the arterial tree to buffer cardiac pulsatility and reflects the mechanical behavior of the vascular parietal system. It represents the return of energy stored by the arterial wall during ventricular ejection.
The τ values obtained were similar to those reported by other authors.21,22 In the present experiments, τ decreased significantly during mechanical occlusion,26 probably due to a considerable reduction in arterial compliance caused by pressure. The non-significant increase in τ during VSM activation (8%) may reflect the balance between the increase in peripheral vascular resistance (pulmonary vasoconstriction) and the decrease in total arterial compliance. The decrease in τ during passive PAH may involve a reduced buffering capacity of the pulsatility of the pulmonary tree, which recovered during active PAH.
The drop in heart rate after phenylephrine infusion (Table 1) may be explained by an increase in vagal discharge caused by the systemic hypertension induced by the phenylephrine.22 To quantify the effects of the cardiac period (T) on τ, the quotient τ/T was calculated for each experimental condition (Table 2). Because the changes in the quotient τ/T were similar to those of τ, the latter is independent of the heart rate during the experimental conditions.
In physiological terms, the pulmonary artery has a large amount of VSM innervated by postganglionic sympathetic fibers that respond to stimulation with significant changes in their mechanical properties.4,5 Thus, sympathetic stimulation or vasoactive substances, such as noradrenaline, nitric oxide and endothelins, may strongly modulate pulmonary artery compliance through acting on VSM tone.21,23 Fitch et al.11 demonstrated that the marked inhibition of nitric oxide synthetase by L-nitro-L-arginine methyl ester increased aortic stiffness (quantified by PWV) to a greater degree than phenylephrine for similar increases in arterial pressure. This indicates that nitric oxide plays a role in the modulation of aortic compliance. Thus, VSM of the great arteries could be seen as a site of physiological modulation of the BF through its effects on parietal elasticity.
According to Poiseuille's law,1,24 mean arterial pressure (stationary component) refers to total peripheral resistance and thus to artery lumen-wall ratio, which means that it affects the conduction function of the arterial system. On the other hand, pulse pressure (pulsatile component) refers to arterial stiffness, and thus to the buffering function of the arterial system. Therefore, during VSM activation, a decrease in pulse pressure was to be expected. Pulse pressure is a complex parameter, and is influenced not only by the ventricular ejection pattern (heart rate and contractility), but also by the arterial parameters of τ, peripheral resistance and total arterial compliance.25,26 Despite BF remaining steady, the significant drop in heart rate may explain the increase in pulse pressure during active PAH compared to baseline conditions. The increase may be due to a longer diastole provoking a greater decrease in diastolic arterial pressure.26 The change in the contraction pattern of the right ventricle due to an increase in afterload, indicated by the synchronization of contraction,14 may also explain the increase in pulse pressure during both active and passive PAH. Finally, the increase in pulsatile diameter during VSM activation demonstrates a greater BF, even with a pulse pressure similar to passive PAH.
Although clinically the hemodynamic characterization of the pulmonary circuit is done by applying Poiseuille' s law (stationary component),1,2,24 the present study establishes a hierarchical characterization of the mechanical behavior of the pulmonary artery in patients with PAH in terms of pulse pressure (pulsatile stress) and pulsatile diameter (linked to compliance and parietal distensibility). This should lead to a better approach to the diagnosis, treatment and prognosis of these patients, including the response to the acute test with vasodilators.
CONCLUSIONS
Moderate and acute pulmonary hypertension modifies the elasticity of the pulmonary artery. When PAH is produced passively without VSM activation (passive PAH), the vascular wall expands, recruiting less-compliant collagen, thus increasing parietal elasticity with the consequent loss of global buffering capacity.
In contrast, when the increase in pulmonary artery pressure involves vascular smooth muscle activation (active PAH), there is a beneficial effect on the arterial mechanism which prevents the increase in parietal stiffness of the pulmonary artery and preserves the global buffering capacity of the pulmonary artery system.
It can be inferred that during active PAH, ventriculo-arterial coupling is preserved at values close to those existing in normal afterload conditions.
The hierarchical study of pulmonary artery wall mechanics suggests the need to define the role of VSM in different clinical categories of PAH.
APPENDIX (TO CALCULATE THE VISCOSITY INDEX)
A 2-component model was used (Kelvin-Voigt viscoelastic model). The total pressure developed by the wall to resist stretching can be split into an elastic component (stored energy) and a viscous component (dissipative energy).17
Given that viscous pressure is proportional to the first derivative of the arterial diameter, equation 2 is expressed as:
where ηp is the viscosity index of the arterial wall and dD/dt is the first derivative of arterial diameter with respect to time. To separate the purely elastic properties of the wall, viscous pressure must be subtracted from total pulmonary pressure, and the optimal value is defined by reducing the area of hysteresis area. The value of ηp was repeteadly increased to reduce the area of hysteresis to a minimum value. The pressure-diameter loop was maintained in a clockwise direction (Figure 1). Once a purely elastic relation was obtained, a logarithmic model previously applied to the description of elastic properties was fitted.10 By exponential transformation, pressure can be expressed as a function of the diameter by two constants, α and β, determined by the fitting procedure:
Assuming that the pulmonary artery wall is made of isotropic elastic material, elasticity (E) was calculated as the slope of the pressure-diameter curve in the mean diastolic pressure with the equation of a purely elastic cylinder with a non-negligible wall.10,12
where h is parietal thickness. Pulmonary parietal thickness was calculated as the difference between the external radius (Re) and the internal pulmonary radius (Ri). The following equation was used to calculate Ri:
where V is the volume and L is the length of the segment of the pulmonary artery wall. During surgery, this segment of the pulmonary artery, which included the piezoelectric crystals, was marked by two sutures, and the distance between them was measured with a gauge. After necropsy, this segment was carefully dissected, cut at the sutures and weighed with a precision balance (Sartorius-Werke type 2442, Germany). Assuming a tissue density of 1066 g/mL, V was calculated from the weight of the segment of the pulmonary artery wall.10,12
Pulse wave velocity was calculated theoretically with the Moens-Korteweg equation.2,10 Our objective was to obtain a measure of parietal stiffness taking into account the geometry of the pulmonary artery:
where hm is the mean parietal thickness, δ is blood density (1055 g/mL) and Rim is the mean internal radius.
The time constant τ was estimated by the best fit of the exponential function in the last third of the diastolic portion of the pulmonary pressure curve, where pulmonary flow is assumed to be zero.18
where t is time and Po is the value of the arterial pressure at time 0 corresponding to the moment of application of the model. The exponential goodness-of-fit was quantified in each condition with the correlation coefficient.
Correspondence: Dr. J.C. Grignola.
Departamento de Fisiología. Facultad de Medicina.
Gral. Flores, 2125. 11800 Montevideo. Uruguay.
E-mail: jgrig@fmed.edu.uy